Commutative Crepant Resolutions
An important problem in algebraic geometry is the resolution of singularities. Given a singular variety, on wants to construct a proper surjective birational map from a smooth variety to the singular one.

There are many ways to do this but one tries to find a resolution that stays as close as possible to the singularity. An example of such resolutions are crepant resolutions and they are compatible with the notion of a canonical divisor.
In dimension 2 a crepant resolution is unique, but in higher dimensions it is not and it might not even exist. Bridgeland showed however that in dimension 3 although there may be different crepant resolutions, they all have the same homological properties. In other words their derived categories of coherent sheaves are equivalent.
Noncommutative Crepant Resolutions
In many cases this derived category is equivalent to the derived category of representations of an algebra. Such an algebra is then called a noncommutative crepant resolution (NCCR). As in the commutative case a singularity can have many NCCRs and in general it is a difficult problem to find these for a given singularity.
If we put some restrictions to this the problem becomes more manageble. If $V$ is a singular toric 3-dimensional gorenstein variety and A is a toric NCCRs (i.e. an NCCR compatible with the toric structure) I proved that A comes from a dimer model on a torus.
Dimers
A dimer model can be seen as a quiver drawn on a torus, such that the arrows go round in cycles around pieces of the torus. The algebra $A$ is then the path algebra of the quiver subject to a set of relations. For every arrow we identify the two paths in opposite direction on the left and the right of the arrow.
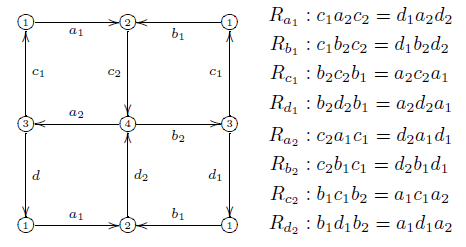
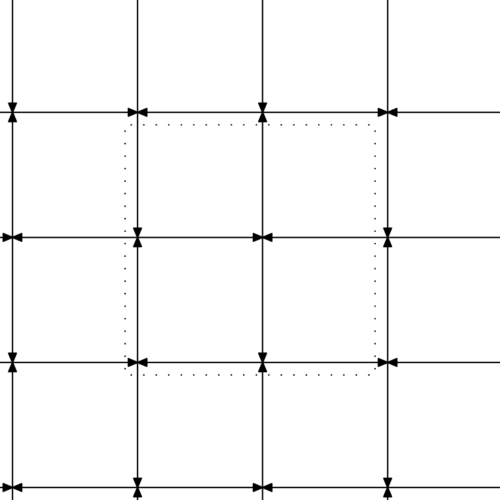
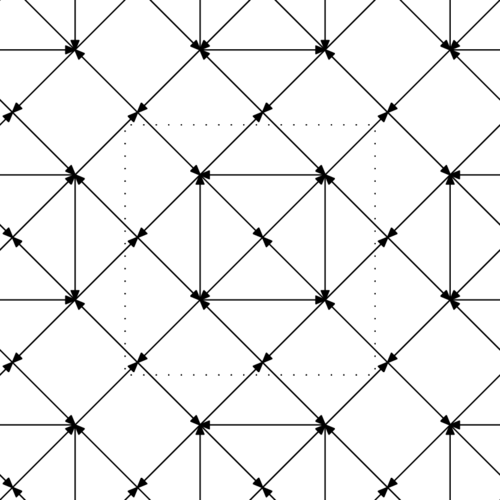
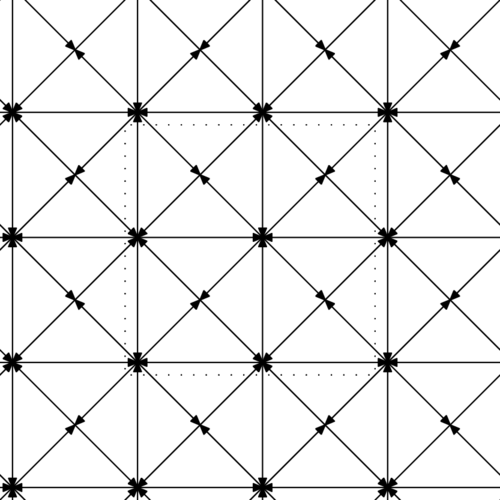
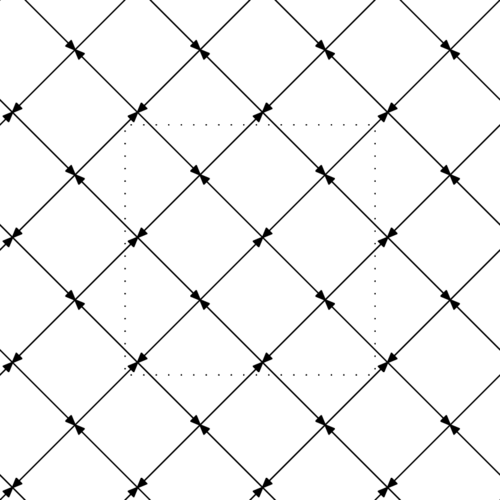
We constructed a program such that for every toric gorenstein 3-singularity spits out all possible dimer models. The toric singularity is determined by the set of ray-vectors of the cone that determines its toric structure as a fan. This data is taken as the input, the output is a set of pictures of the dimer model. The dimer model is pulled back to the universal cover of the torus and gives us a periodic quiver. The fundamental domain is drawn as a dotted square. So for the dimer above you get this picture.
Fish tilings
Below we give an example of the singularity with as coordinate ring the subring of C[X,Y,Z] spanned by the
monomials XaYbZc with ∓a ∓b+c ≥0.
The rays are given by the coefficients of the inequalities:
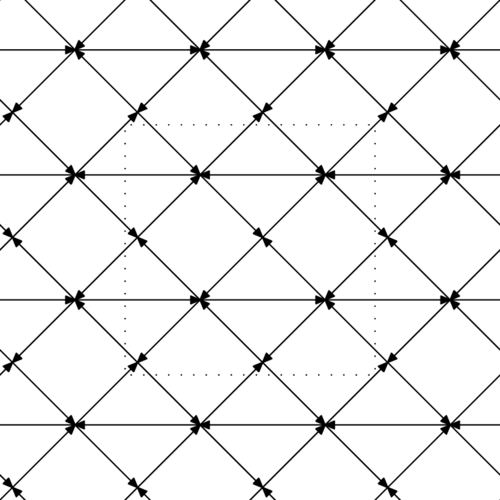
There are 4 dimer models. For each we give irs quiver.
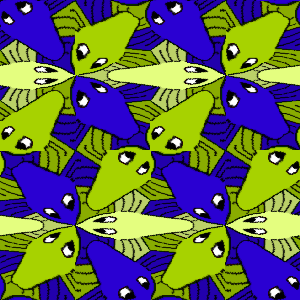
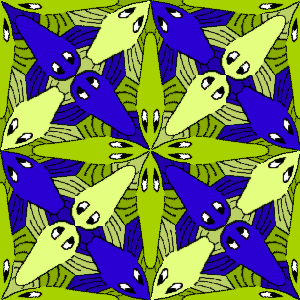
For the more artistically minded we also transformed the quiver to a tiling of the plane with fishes. Each fish represents an arrow in the quiver from its tail to its head.
|
|
|

|
|
|
|
|