Research highlights

Quantum turbulence without energy cascade

Experiments and numerical simulations of turbulent superfluid helium have established that, at hydrodynamic length scales larger than the average distance between quantum vortices, the energy spectrum obeys the same 5/3 Kolmogorov law which is observed in the homogeneous isotropic turbulence of ordinary fluids. The importance of the 5/3 law is that it points to the existence of a Richardson cascade which transfers kinetic energy from large eddies to small eddies. However, there is also experimental evidence of quantum turbulent regimes without Kolmogorov scaling. Why does the Kolmogorov spectrum fail to form in such regimes? What is the physical nature of turbulence without energy cascade ?

In this work we describe simple physical mechanisms which prevent the formation of Kolmogorov scaling in superfluid helium at both high and low temperatures. Thee first regime, at high temperatures, is turbulence generated by a heat flux (thermal counterflow). The second regime, at low temperatures, is turbulence generated by injecting vortex rings.

CF Barenghi, YA Sergeev, and AW Baggaley, Regimes of turbulence without an energy cascade' Nature Scientific Reports 6 35701 (2016), (arXiv) (article)

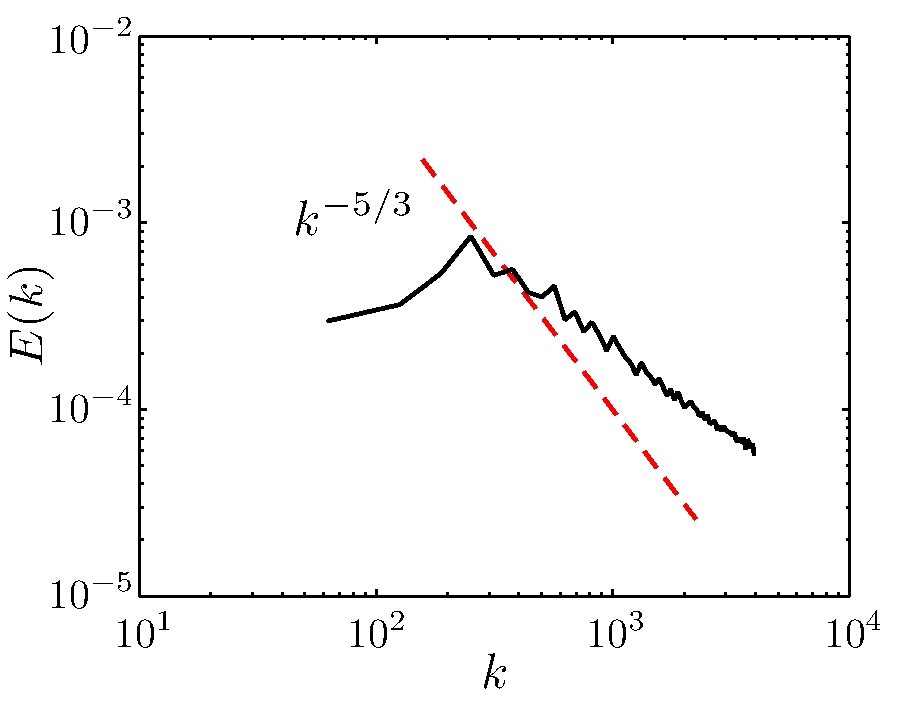
Computed superfluid energy spectrum E(k) vs wavenumber k for thermal conterflow. Note that energy is concentrated at intermediate k (not at small k as in classical turbulence) and, at large k, differs from the classical k^(-5/3) Kolmogorov scaling (red dashed line).

Leapfrogging vortex rings and Kelvin waves

Two coaxial vortex rings at close distance to each other form a "leapfrogging" configuration (the vortex which is initially behind shrinks, speeds up, goes inside the vortex which was initially in front, overtaling it, then becomes larger and slows down; at this point the process repeats, without any reconnection, without ending).

This phenomenon has been known since the times of Helmoltz, and has been recently generalized (DH Wacks, AW Baggaley and CF Barenghi, Physics of Fluids, 26, 027102, 2012) to configurations of up to about 100 vortex rings and compared to experiments. For example, the left figure shows three leapfrogging vortex rings.

Using again parameters suitable for superfluid helium, here we describe a recurrence phenomena similar to leapfrogging rings which occurs for two coaxial straight vortex filaments with a helical perturbation (called Kelvin wave). The figure at the right shows a vortex initially inside a helical Kelvin wave; the inner vortex develops a Kelvin wave of the same wavelenght which grows in amplitude, so that the inner vortex becomes the outer vortex, and the process repeats periodically without any reconnection. Besides superfluids, helical vortices are also important in viscous flows, where they appear in wakes behind propellers.

Niklas Hietala, Risto Hanninen, Carlo F Barenghi and Hayder Salman, Leapfrogging Kelvin waves to appear in Phys Rev Fluids (2016), (arXiv)

DH Wacks, AW Baggaley and CF Barenghi, Coherent laminar and turbulent motion of toroidal vortex bundles, Phys of Fluids, 26 027102 (2012), (arXiv) (article)

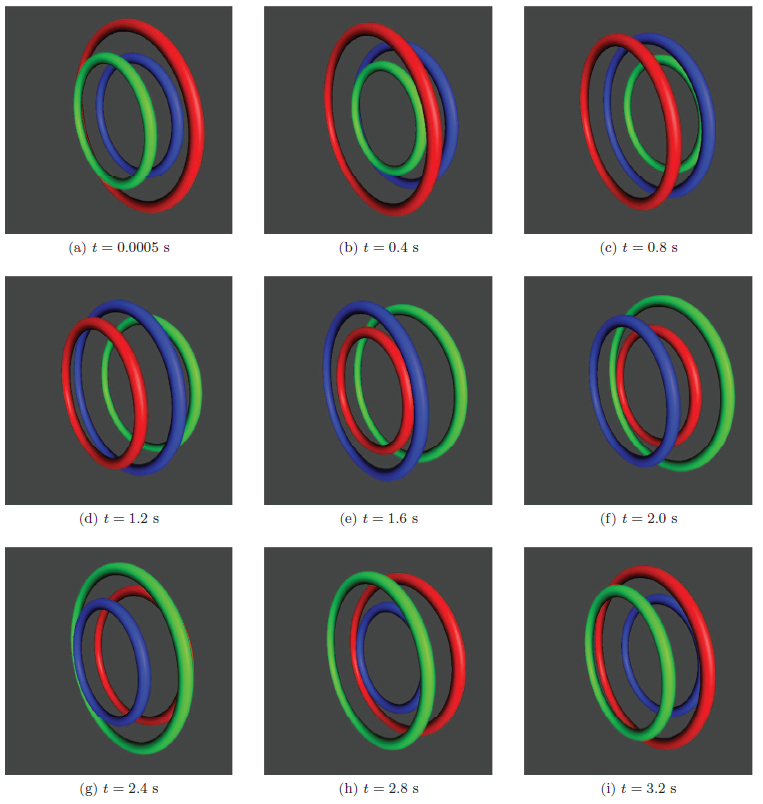
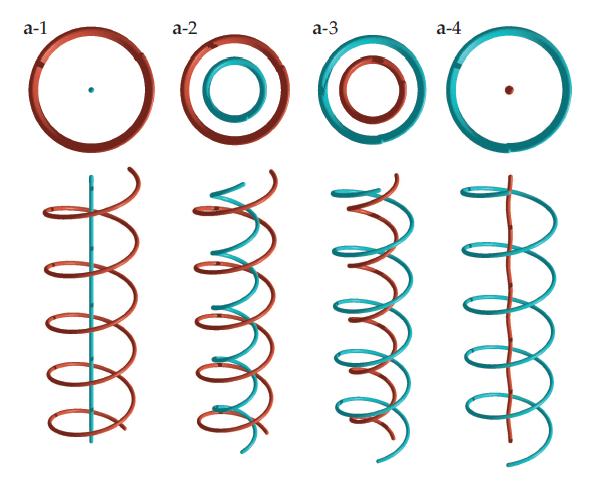
Left: Three leapfrogging vortex rings (Phys Fluids 2012); notice for example the red vortex which is initially ahead and which grows in size, slowing down and falling behind the other rings, and then overtakes them again from the inside. Right: Leapfrogging Kelvin waves (Phys Rev Fluids to be published), top and side views. Notice the blue vortex, initially straight and inside the red vortex with Kelvin wave, which develop a Kelvin wave of the same wavelength; this Kelvin wave grows in amplitude until the blue vortex becomes the outer vortex and the red vortex becomes the inner vortex.

Ultra-quantum turbulence in a quenched homogeneous Bose gas

Using the classical field method, we study numerically the characteristics and decay of the turbulent tangle of quantum vortices which is created in the evolution of a Bose gas from highly nonequilibrium initial conditions. By analysing the vortex line density, the energy spectrum and the velocity correlation function, we determine that the turbulence resulting from the thermal quench lacks the coherent structures and the Kolmogorov scaling (typical of ordinary turbulence and of turbulent superfluid helium when driven by grids or propellers). Instead, thermal quench turbulence has properties akin to a random flow, more similar to another turbulent regime called ultra-quantum turbulence which has been observed in superfluid helium.

GW Stagg, NG Parker and CF Barenghi, Ultra-quantum turbulence in a quenched homogeneous Bose gas to appear in Phys Rev A (2016)

arXiv

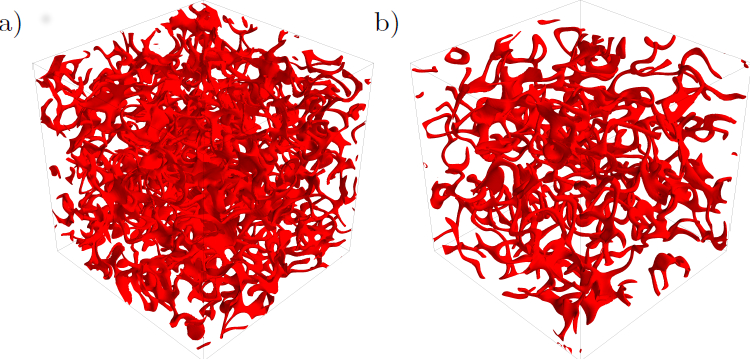
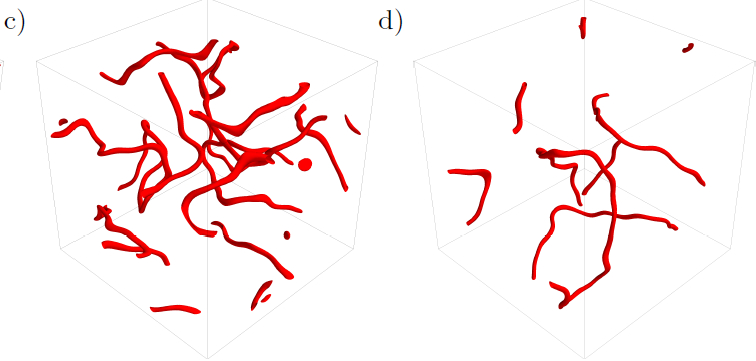
Formation and decay of turbulent tangle of quantum vortices. At t=0 the occupation number n(k) is uniformly distributed in k-space. Quickly, n(k) concentrates at low k (large length scales) creating a condensate. Phase defects organize into a turbulnt tangle of vortices, which slowly decays to a vortex-free state)

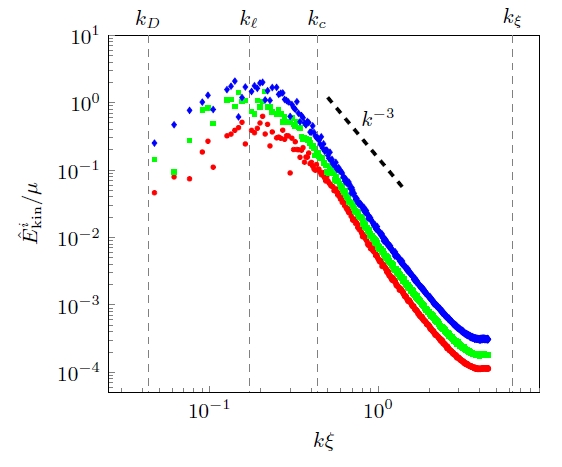
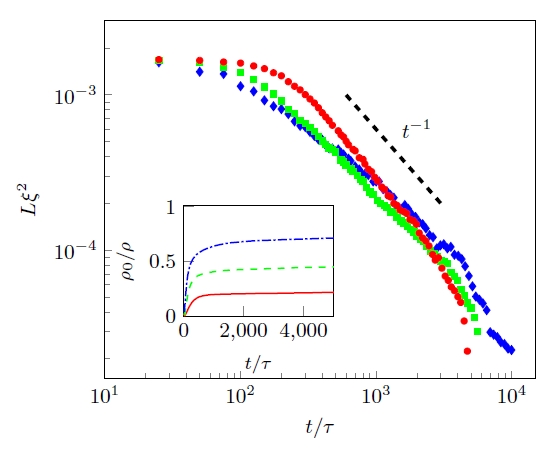
Left: energy spectrum. Note that energy peaks at the intermediate length scales (rather than the largest length scales (small k). Right: temporal decay of the vortex line density L ; note that L~t^(-1).

Quantum turbulence in trapped atomic Bose Einstein condensates

Turbulence, the complicated fluid behavior of nonlinear and statistical nature, arises in many physical systems across various disciplines, from tiny laboratory scales to geophysical and astrophysical ones. The notion of turbulence in the quantum world was conceived long ago by Onsager and Feynman, but the occurrence of turbulence in ultracold gases has been studied in the laboratory only very recently. Albeit new as a field, it already offers new paths and perspectives on the problem of turbulence.

Here we review the general properties of quantum gases at ultralow temperatures paying particular attention to vortices, their dynamics and turbulent behavior. We review the recent advances both from theory and experiment. We highlight the difficulties of identifying and characterizing turbulence in gaseous Bose-Einstein condensates compared to ordinary turbulence and turbulence in superfluid liquid helium and spotlight future directions of research.

MC Tsatsos, PES Tavares, A Cidrim, AR Fritsch, M Caracanhas, FE dos Santos, CF Barenghi, and VS Bagnato, Quantum turbulence in trapped atomic Bose-Einstein condensates' Physics Reports 622 1-52 (2016)

arXiv article

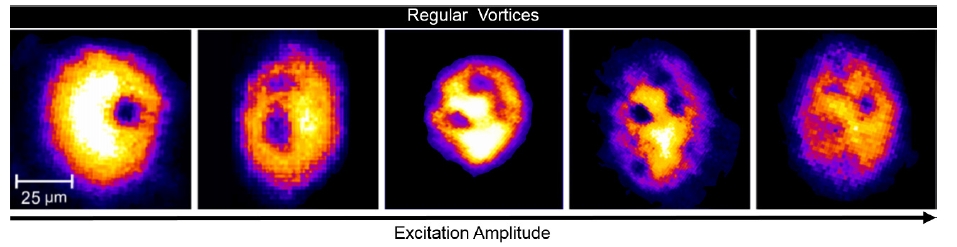
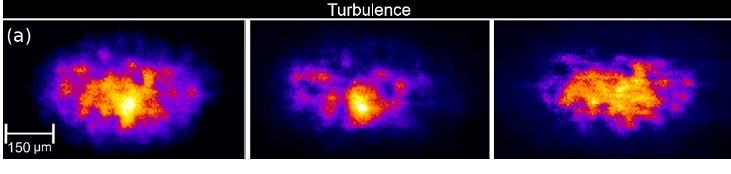
Top: experimental absorption images of condensates containing 1, 2, 3 and more quantum regular vortices. Bottom: images of condensates containing turbulent vortices.

Visualizing pure quantum turbulence in superfluid He3

Superfluid He3-B at very low temperatures gives insight into quantum turbulence in its most pure and fundamental form - a tangle of vortex filaments in a fluid without viscosity which move under the influence of each vortex on all the others (Euler dynamics) and also reconenct with each other. At these extreme low tempertures (175 microKelvin) flow visualization is not trivial: it is based on Andreev scattering of ballistic thermal excitations, a peculiar scattering in which a quasiparticle is bounced off the velocity field of a quantum vortex, becoming a quasiholes and (unlike classical reflections) going back along the same direction it arrived (the same scattering affects incoming quasiholes).

In this work we show the relation between the vortex line density (which characterizes the intensity of the turbulence) and the Andreev reflectance of the vortex tangle, in the first simulations of Andreev reflections by a realistic turbulent tangle of vortices. We compare the results with experiments, probing for the first time the turbulence on length scales smaller than the vortex separation.

AW Baggaley, V Tsepelin, CF Barenghi, SN Fisher, GR Pickett, YA Sergeev, and N Suramlishvili, Visualizing Pure Quantum Turbulence in Superfluid 3He: Andreev Reflection and its Spectral Properties Phys. Rev. Lett. 115, 015302 (2015)